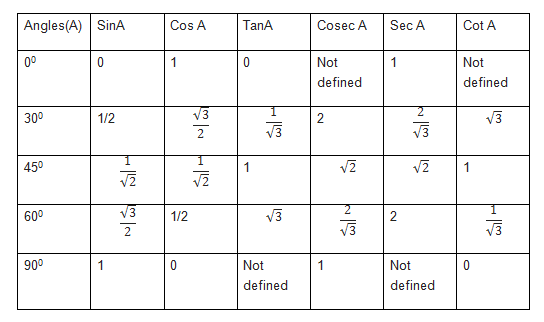
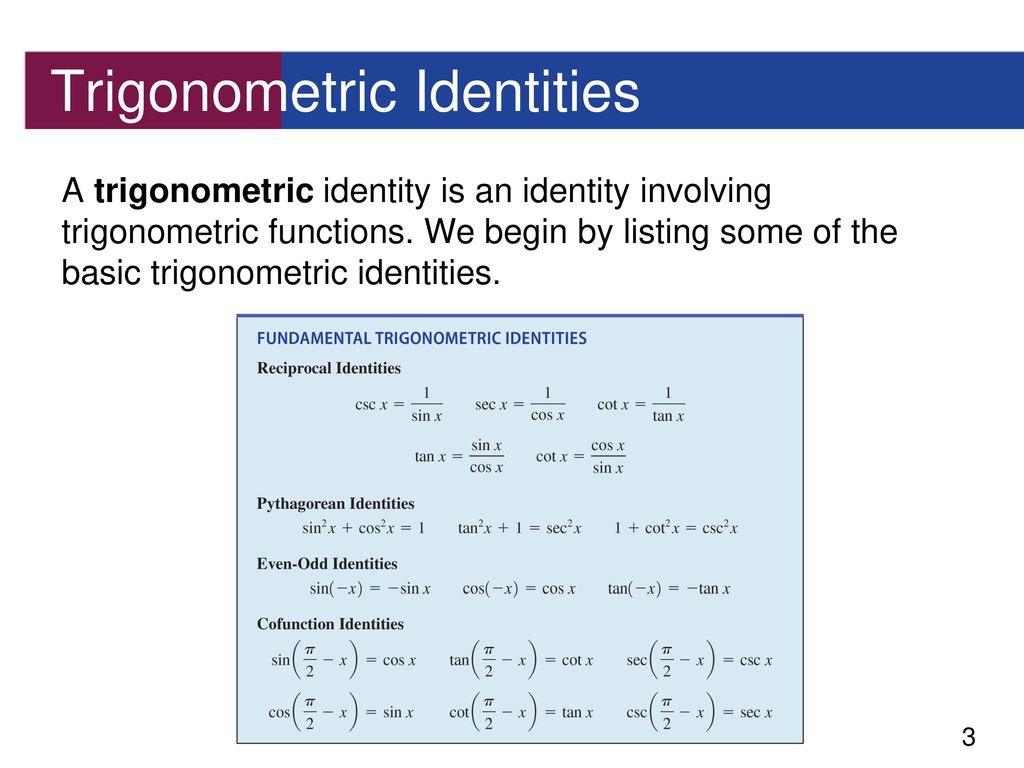
Trigonometric Identities - ppt download
Trigonometric Ratios of (180 ) y The circle is centred at the origin O. It cuts the positive x-axis at A. A x O
y. The circle is centred at the origin O. It cuts the positive x-axis at A. A. x. O.
y. P(3, 4) is a point on the circle. P(3, 4) AOP = A. x. O. By the definitions of trigonometric ratios, sin = cos = tan =
y. P is reflected about the y-axis to Q. Q(3, 4) Q. P(3, 4) Coordinates of Q = (3, 4) 180º - q. A. x. O. AOQ = 180 By the definitions of trigonometric ratios, sin (180 ) = cos (180 ) = tan (180 ) =
tan = tan (180 ) = sin (180 ) = sin cos (180 ) = cos tan (180 ) = tan
In fact, the relationships between the trigonometric ratios of and (180 ) are true for any acute angle .
Suppose P(a, b) is a point on a circle of radius r centred at the origin O. y. P(a, b) By the definitions of trigonometric ratios, r. B. A. x. O. sin = r. b. cos = r. a. tan = a. b.
Q(a, b) Q. P(a, b) r. r. Coordinates of Q = (a, b) 180º – q. B. A. x. O. OQ = r. AOQ = 180
Q(a, b) P(a, b) sin (180 ) = r. b. = sin r. 180º – q. B. A. x. O. r. a. cos (180 ) = - = -cos a. b. tan (180 ) = - = -tan cos = r. a. tan = b. sin = Note:
sin (180 ) = sin cos (180 ) = cos tan (180 ) = tan If is an acute angle, then (180 ) lies in quadrant II, and only sin (180 ) is positive.
y. R is obtained by rotating P(a, b) through 180 about O. P(a, b) r. 180º + q. 180 Coordinates of R. = (a, b) A. x. O. OR = r. r. Reflex AOR. = 180 + R(a, b) R.
y. By the definitions of trigonometric ratios, P(a, b) sin (180 + ) = r. b. = -sin 180º + q. A. x. O. r. a. cos (180 + ) = = -cos r. R(a, b) a. b. tan (180 + ) = = tan cos = r. a. tan = b. sin = Note:
sin (180 + ) = –sin cos (180 + ) = –cos tan (180 + ) = tan If is an acute angle, then (180 + ) lies in quadrant III, and only tan (180 + ) is positive.
y. S is obtained by reflecting P(a, b) about the x-axis. P(a, b) Coordinates of S = (a, b) r. 360º - q. A. x. OS = r. O. q. r. Reflex AOS. = 360 S. (a, b)
y. By the definitions of trigonometric ratios, P(a, b) sin (360 - ) = r. b. - = -sin 360º - q. A. x. O. r. a. cos (360 - ) = = cos r. S. (a, b) a. b. tan (360 - ) = = -tan - cos = r. a. tan = b. sin = Note:
sin (360 – ) = –sin cos (360 – ) = cos tan (360 – ) = –tan If is an acute angle, then (360 – ) lies in quadrant IV, and only cos (360 – ) is positive.
What do you observe about the terminal sides of and (360 )
y. 360 - x. O. - The terminal sides of - and (360 - ) are coincident.
sin (360 – ) = –sin cos (– ) = cos (360 – ) = cos tan (– ) = tan (360 – ) = –tan If is an acute angle, then – lies in quadrant IV, and only cos (– ) is positive.
x. y. 360 + The terminal sides of and (360 + ) are coincident. We have. sin (360 + ) = sin cos (360 + ) = cos tan (360 + ) = tan
Let’s summarize using the ‘CAST’ diagram.
Sine is positive. All are positive. O. y. x. S. A. sin (180 – ) = + sin q. sin (360 + ) = + sin q. cos (180 – ) = – cos q. 180 - cos (360 + ) = + cos q. 180 + 360 - tan (180 – ) = – tan q. tan (360 + ) = + tan q. T. C. sin (180 + ) = – sin q. sin (360 – ) = – sin q. cos (180 + ) = – cos q. cos (360 – ) = + cos q. tan (180 + ) = + tan q. tan (360 – ) = – tan q. Tangent is positive. Cosine is positive. These identities are also true even if is not an acute angle.
Express each of the following trigonometric ratios in terms of the same trigonometric ratio of an acute angle. (a) cos 125 = cos (180 55) = cos 55 cos (180 ) = cos
Express each of the following trigonometric ratios in terms of the same trigonometric ratio of an acute angle. (b) sin (250) = sin [360 + (250)] sin (360 + ) = sin = sin 110 = sin (180 70) = sin 70 sin (180 ) = sin
Find the values of the following trigonometric ratios. (a) sin 210 = sin (180 + 30) = –sin 30 sin (180 + ) = sin =
Find the values of the following trigonometric ratios. (b) tan 315 = tan (360 – 45) = –tan 45 tan (360 ) = tan = –1.
Follow-up question Simplify .
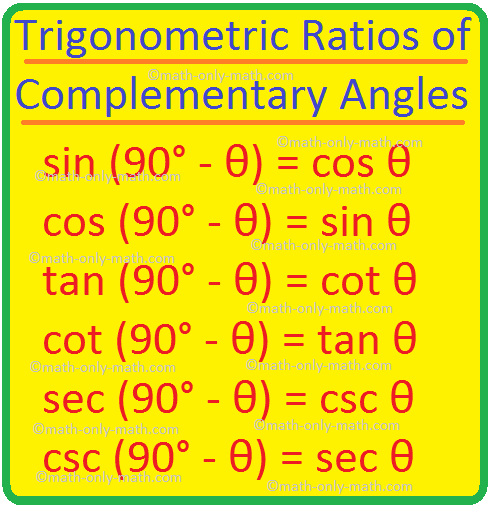
By using the trigonometric ratios of (180 - q) and (90 - q), we have. ) 90 sin( q. + ) 90 180 sin( q. + - = )] 90 ( 180 sin[ q. - = ) 90 sin( q. - = sin (180 - f) = sin f. q. cos. = ) 90 cos( q. + ) 90 180 cos( q. + - = )] 90 ( 180 cos[ q. - = ) 90 cos( q. - = cos (180 - f) = -cos f. q. sin. - =
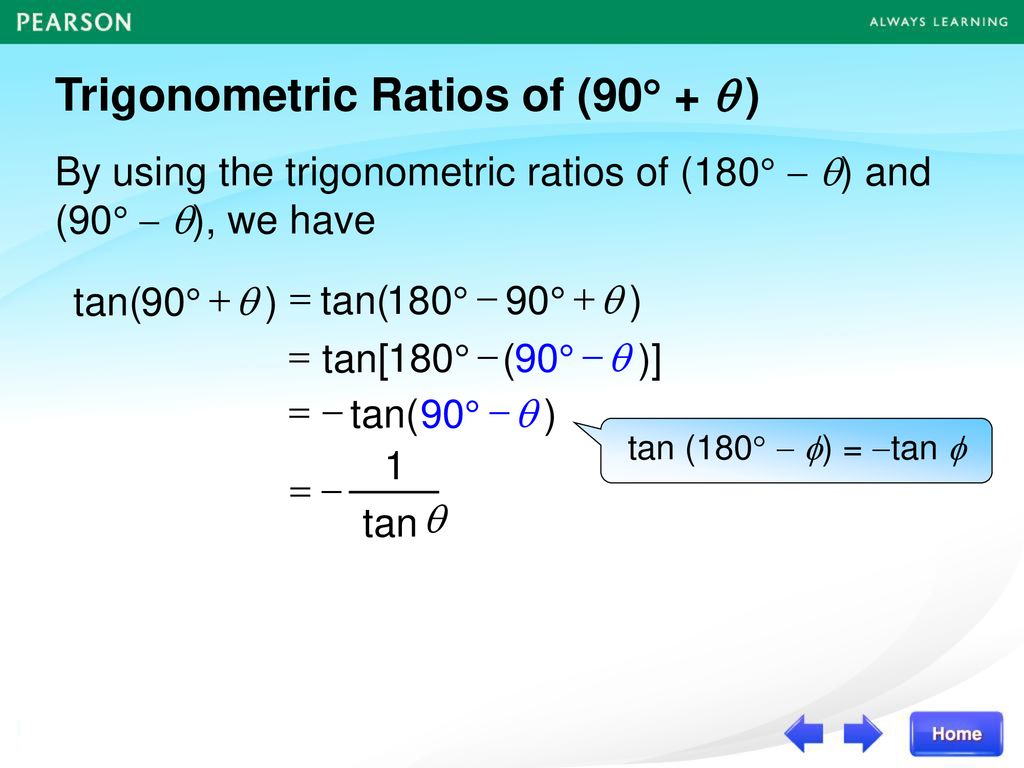
By using the trigonometric ratios of (180 - q) and (90 - q), we have. ) 90 tan( q. + ) 90 180 tan( q. + - = )] 90 ( 180 tan[ q. - = ) 90 tan( q. - = tan (180 - f) = -tan f. q. tan =
sin (90 + ) = cos cos (90 + ) = -sin tan (90 + ) = These identities are also true even if is not an acute angle.
By using the trigonometric ratios of (180 + ) and (90 - q), we have. sin (270 – q) = sin [180 + (90 – )] = –sin(90 – ) = –cos sin (180 + f) = –sin f. cos (270 – q) = cos [180 + (90 – )] = –cos (90 – ) = –sin cos (180 + f) = –cos f.
By using the trigonometric ratios of (180 + ) and (90 - q), we have. tan (270 – q) = tan [180 + (90 – )] = tan (90 – ) q. tan. 1. tan (180 + f) = tanf. =
By using the trigonometric ratios of (360 – ) and (90 – q), we have. sin (270 + q) = sin [360 – (90 – )] = –sin (90 – ) = –cos sin (360 – f) = –sin f. cos (270 + q) = cos [360 – (90 – )] = cos (90 – ) = sin cos (360 – f) = cos f.
By using the trigonometric ratios of (360 – ) and (90 – q), we have. tan (270 + q) = tan [360 – (90 – )] = –tan (90 – ) tan (360 – f) = –tanf. q. tan. 1. – =
sin (270 - ) = -cos sin (270 + ) = -cos cos (270 - ) = -sin cos (270 + ) = sin tan (270 - ) = tan (270 + ) =
Sine is positive. All are positive. O. y. x. S. A. sin (90 + ) = + cos q. sin (90 – ) = + cos q. cos (90 + ) = – sin q. cos (90 – ) = + sin q. 90 + 270 – 270 + tan (90 + ) = – 90 – tan (90 – ) = + T. C. sin (270 – ) = – cos q. sin (270 + ) = – cos q. cos (270 – ) = – sin q. cos (270 + ) = + sin q. tan (270 – ) = + tan (270 + ) = – Tangent is positive. Cosine is positive. These identities are also true even if is not an acute angle.
Follow-up question Simplify . cos q sin q tan =

Unit 3: Trigonometric Identities - ppt video online download

Trigonometric Identities - ppt download
3.6.1 Simple Identities DP IB Maths: AA HL Revision Notes 2021

Trigonometric identities

5.1 Using Fundamental Identities. Fundamental Trigonometric Identities. - ppt download
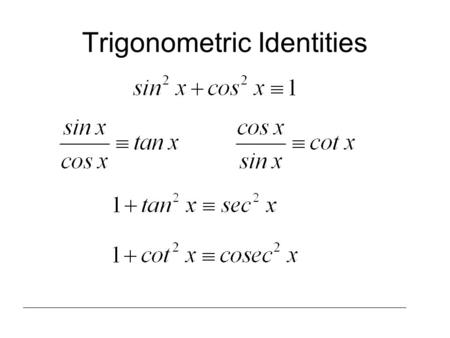
Basic Trigonometric Identities - ppt download

7.2 Verifying Trigonometric Identities - ppt download
Trigonometric Identities - ppt download

Topic 3: Circular Functions and Trigonometry - IB DP Mathematics SL

Reciprocal Identities - FasterCapital

EXAMPLE 4 Graph a translated square root function Graph y = –2 x – 3 + 2. Then state the domain and range. SOLUTION STEP …